What's All This Algebraic Equation Stuff, Anyhow? (Part 1)

Check out More Bob Pease on Analog.

Download the "What's All This Algebraic Equation Stuff, Anyhow?" eBook.

Article updated 10/04/22

• Part 1
• Part 2
• Part 3

You guys may have noticed that recently, I have been showing you a lot of algebraic equations. I really don't like to use, or generate, algebraic equations. Sometimes they're much messier than just doing numerical evaluations.

For example, to evaluate the response of a filter, I usually like to plug in the R and C values, and simplify as much as possible before I do the full analysis. I just prefer to get the numbers into number form as early as possible. But sometimes to generalize properly, it really makes sense to write down an equation.

For instance, I gave you the Wye-Delta and Delta-Wye transforms in "Tee Network Stuff" (Electronic Design, June 4, p. 113). I listed a big equation in "V_BE Stuff" (Electronic Design Analog Supplement, June 26, 2000, p. 15) and set up some equations in "Transimpedance Amplifier Stuff" (Electronic Design, Jan. 8, p. 139). So it's not that I can't do algebraic equations—I just prefer not to, usually.

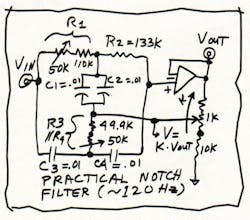
Recently, I had planned on going to an excellent museum. But I wanted to correctly finish the derivation of an equation that had been giving me trouble and wasting a lot of my time, as I had obviously been making errors. This just happens to be the circuit shown in the figure.

I knew what the solution had to be like, but I was getting stupid answers and messy equations that made no sense. So I knew that I had to stop making dumb errors. (The solution I had didn't even have the right dimensions.) I knew the solution had to look very much like:

    V_OUT = V_IN × [1 + 0·pRC + (pRC)²] / [1 + K1·pRC + (pRC)²]

where K1 is some function of k, and pC is the reciprocal of the impedance of a capacitor: pC = j × 2πfC. We also are going to assume that C1 = C2 = C3 = C4 = C, exactly, and that R1 = R2 = R3 = R4 = R, exactly. (Later, we will discuss how to fix things if the Rs and Cs aren't perfect.) We're assuming that the gain and CMRR of the amplifier are plenty high too (and that the gain errors and CMRR errors are small enough and linear enough) at the frequencies you're interested in. Or, if they're not quite small, we will assume we can trim them out.

This works much better than most forms of notch filter. If you need one, this is worth building. I have some other tricks up my sleeve, too. So it's not that I can't do algebraic equations, I just prefer not to—usually. But when it's important, I know how to do them, and I can show you how as well.

I sat in my car in the museum's parking lot, sipping some beer and crunching along on about seven pages of scrap paper. Finally, I came up with the right answer. The above formula was exactly right, and the correct function for K1 turned out to be: K1 = 4 (1 −k).

This means that if k is very close to 1, K1 gets very small, and the Q of this filter is very high (very good). This is much easier to see, in the general case, by using the algebraic equations than by just plugging in the component values. It means that if you have gotten a good notch filter, with the right depth and the right center frequency, you can use the pot at k to trim to get any Q you want without significant interaction. Next time, I will show you how to do the math.

All for now.

Read Part 2 now.

This article was published in Electronic Design Oct 1, 2001

see Bob Pease Remembered — One Year Later

This is how Bob always ended his blog. The information is no longer valid. 

/ Comments invited!
RAP / Robert A. Pease / Engineer
[email protected]—or:

Mail Stop D2597A
National Semiconductor
P.O. Box 58090
Santa Clara, CA 95052-8090