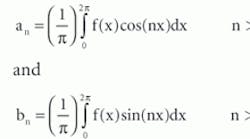Modified Sine-Wave Inverter Enhanced
With the increasing popularity of alternate power sources, such as solar and wind, the need for static inverters to convert dc energy stored in batteries to conventional ac form has increased substantially. Most use the same basic concept: a dc source of relatively low voltage and reasonably good stability is converted by a high-frequency oscillator and stepup transformer to a dc voltage with magnitude corresponding to the peak of the desired ac voltage. A power stage at the output then generates an ac voltage from the higher-voltage dc. Conceptually, the operation is illustrated in Fig. 1.
Current State of the Art
There are basically two kinds of dc-ac inverters on the market today. One category is the “pure sine-wave” inverter, which produces sine waves with total harmonic distortion (THD) in the range of 3% (-30 dB). These are typically used when there is a need for clean, near-sine-wave outputs for medical, instrument and other critical applications.
Some, for example, are used in boats and RVs as the main source of electricity, and some feed energy back into the utility power grid. Waveforms approaching sine waves, with minimal distortion, are required in any case. These inverters are available in sizes up to several thousand watts and typical costs are in the range of $0.50 per watt (visit invertersrus.com for an example). Early techniques for designing these true sine-wave inverters incorporated significant linear technology, reducing their efficiency and contributing to their higher cost.
More recent designs used pulse-width modulation (PWM) to produce a pulsed waveform that can be filtered relatively easily to achieve a good approximation to a sine wave (for example, see U.S. patent numbers 4,742,441; 4,600,984; 6,980,450; and 4,466,052). The significant advantage of the PWM approach is that switching techniques are used in the power stages, resulting in relatively high efficiency.
However, PWM, with the pulse width made to vary according to the amplitude of a sine wave, requires significant control circuitry and high-speed switching. This is because the frequency of the PWM signal has to be much higher than that of the sine wave to be synthesized if the PWM signal is to be filtered effectively. So the PWM approach introduces significant complexities and switching losses.
The second category consists of relatively inexpensive units, producing modified sine-wave outputs, which could logically be called “modified square waves” instead. They are basically square waves with some dead spots between positive and negative half-cycles. Switching techniques rather than linear circuits are used in the power stage, because switching techniques are more efficient and thus less expensive. These inverters require no high-frequency switching, as the switching takes place at line frequency. Their costs are generally in the range of $0.10 per watt (for an example, see www.invertersrus.com/inverters.html).
The typical modified sine-wave inverter has a waveform as shown in Fig. 2. It is evident that if the waveform is to be considered a sine wave or a modified sine wave, it is a sine wave with significant distortion.
Analysis of Current Technology
It is well known that any periodic waveform such as that mentioned previously can be represented by a Fourier series, an infinite sequence of sines and cosines, at the fundamental frequency of the waveform and its harmonics. These harmonics can cause trouble in several areas — particularly in motors and sensitive applications — and the data sheets for the inverters frequently caution the user that certain devices may not work with the inverter. Furthermore, even though the root-mean-square (RMS) value of the waveform may be a nominal 115 V or 120 V, the peak will be different than that of a true sine wave, and that factor can cause trouble in applications that depend on the peak value.
The actual percent distortion is not usually quoted in the specifications for inverters other than the pure sine-wave versions, so it is instructive to compute the distortion products to get a feel for the relative distortion involved with the different approaches. For purposes of comparison, let us look first at a conventional square wave (Fig. 3). The coefficients of the Fourier series are computed with a pair of integrals that produce the coefficients of the sine and cosine terms in the series.
For a signal f(x) with a zero dc component, the integrals are:
where the an and bn terms are the coefficients of the cosine and sine terms, respectively, in the series. The Fourier series is then:
f(x) = a1cos x + a2cos 2x + a3cos 3x + … + b1sin x + b2sin 2x + b3sin 3x + …
The complete background on Fourier series, as well as treatment of special cases, is covered in several textbooks on networks or engineering mathematics, and will not be repeated here. We will just note that because both the square wave and the modified sine wave have both half-wave symmetry and quarter-wave symmetry, integration is required only over one-quarter of the waveform, and further that only the sine terms and odd harmonics are required. Thus, the integral used to compute the coefficients for the conventional square wave becomes:
The series is then (4/π)sin x + (4/3π)sin(3x)+(4/5π)sin(5x) + …
The standard measure of distortion is THD defined as:
Numerical evaluation of the coefficients for the square wave indicates that if the square wave is to be considered a sine wave with distortion, the THD is in the range of 45% (-7 dB). The third harmonic, the hardest to filter out, is one-third the magnitude of the fundamental (-10 dB).
Turning now to the modified sine wave, let us define the width of the positive and negative portions as 2α as depicted in Fig. 4. Again noting that the waveform has both half-wave symmetry and quarter-wave symmetry, and carrying out the integration from 0 to π/2, we have:
Evaluation of this expression for various values of a indicates that the minimum harmonic distortion occurs at α = 0.352π, where the THD is 23.8% (-12 dB), about half that of the square wave. The third harmonic is about 6.5% (-24 dB) of the fundamental, also a significant improvement over the square wave. However, these figures indicate that the modified sine wave is far from being a true sine wave, and suggest that improvement is in order.
Proposed Improvement
Consider now a further modification — the addition of another level. The waveform is shown in Fig. 5. Again using the fact that the waveform has both half-wave and quarter-wave symmetry, we carry out the integration over the period 0 to π/2, with the result that:
This result has four variables, of which all could theoretically be varied to achieve minimum distortion. However, one particularly efficient approach is to choose a very simple set of values for A and B — namely B = 2×A — and then optimize the values of a and b for minimum distortion. This approach requires only two positive and two negative power-supply voltages, all of which can be generated from a single transformer in the high-frequency oscillator. (Other values of A versus B may be useful, but were not investigated because the simple relationship of B = 2×A had very good results as discussed later.)
With this restriction, evaluation of the Fourier coefficients indicates that the minimum distortion that can be achieved is about 6.5% (-24 dB), and occurs at β = 0.42π and α = 0.248π. The third harmonic is only about 0.17% (-55 dB) of the fundamental, suggesting that minimal low-pass filtering would greatly reduce the fifth and higher-order harmonics and produce a relatively clean sine wave. The third harmonic can be eliminated entirely, with β = 0.42π and α = 0.246698π, at the expense of slightly higher THD.
[Note: The Fourier analysis was carried out through the ninth harmonic for all three types of waveforms considered in this article. Harmonics above the ninth are not negligible, but any filtering applied to reduce the third through ninth harmonics will be even more effective on those above the ninth. Therefore, the higher-order harmonics are ignored in this analysis.]
Implementation
As demonstrated here, the modified-sine-wave inverter can be modified further to produce a much closer approximation to a sine wave, at a relatively small increase in manufacturing costs, simply by incorporating another level into the waveform. The design still uses switching technology in the power stage, assuring high efficiency. A patent application has been submitted for the approach described in this article.
The switching stage could be implemented with a combination of bridge and half-bridge components commonly used in power switching applications. To produce the proposed multiple-level waveform, several implementations are possible. In general, they all involve connecting the output lead to a specific voltage level with switches such as power MOSFETs capable of handling substantial current. Consider the block diagram shown in Fig. 6 where the voltages A and B correspond to the voltage levels defined previously.
Appropriate digital logic and timing circuits will be used to activate each switch at the correct time to achieve the α and β pulse widths. A table can be developed to indicate which switches must be closed for each section of the output waveform. Note that Switch #3 in Fig. 6 will need to be a bidirectional switch, since it must switch the output lead VOUT to ground regardless of any voltage present in the load. All other switches can be unidirectional.
Unlike conventional PWM-inverter designs, which switch at high frequencies, the proposed inverter design switches at just three times the line frequency. As a consequence, the proposed inverter design will reduce switching losses from that of the PWM-controlled inverter and will save power regardless of the output power level.
For further details on implementation, contact the author at [email protected].