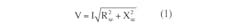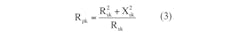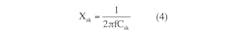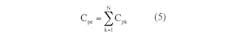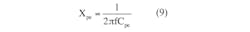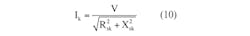Determine Equivalent ESR, Ripple Voltage, And Currents For Unequal Capacitors In Parallel

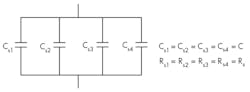
If all N capacitors in the parallel connection are identical (Fig. 1), with equal capacitance values C_sk = C and equal ESR values R_sk = R_s, then for k = 1, 2, . . . N the answers are clear:

• C_se is directly proportional to the number of capacitors N: C_se = NC, and R_se is inversely proportional to N: R_se =R_s/N. 

• Ripple voltage V (RMS value) is:

for a sinusoidal current excitation i(t) = I √2 sin (2πft) with frequency f, where X_se = 1/(2πfC_se) is the reactance of the equivalent capacitor C_se and RMS value I, and individual RMS currents in the capacitors are identical: I_k = I/N.

When the capacitors in the parallel connection aren’t identical, with different capacitance C_sk and ESR R_sk values, the solution to the problem isn’t trivial. The direct approach is to obtain an analytical expression for the input impedance of the parallel connection in the algebraic form Z = Re Z – j ImZ=  Z_se Z and use the formulas R_se = Re Z, X_se = Im Z, and C_se = 1/(2πf X_se).

A less complicated approach is based on the conversion of series C_sk,R_sk connections to equivalent parallel C_pk, R_pk connections. To obtain relationships between R_pk and R_sk, and also between C_pk and C_sk, set the admittance Y_pk of the parallel C_pk, R_pk pair and admittance Y_sk of the series C_sk, R_sk pair connections equal to each other: Y_pk = Y_sk, Re (Y_pk) = Re (Y_sk), and Im (Y_pk) = Im (Y_sk). Then:

where:

is the reactance of the individual capacitor.

After individual parallel capacitances C_pk and resistances R_pk are calculated according to Equations 2 and 3, equivalent parallel capacitance C_pe can be easily found as the sum of C_pk:

The real part of equivalent admittance can be found as the sum of admittances 1/R_pk. R_pe can be obtained as a reverse value of that sum:       

The system’s equivalent series capacitance C_se and ESR R_se  can be found by conversion of the parallel C_pe, R_pe connection to the equivalent series connection C_se, R_se. To obtain relationships between C_se and C_pe and also between R_se and R_pe, set impedance Z_pe of the parallel C_pe, R_pe and impedance Z_se of the series C_se, R_se connections equal to each other: Z_pe =  Z_se, Re Z_pe =Re Z_se, Im Z_pe =  Im Z_se. Then:

where:

is the reactance of the equivalent parallel capacitor C_pe(Equation 5).

Based on this analysis, the calculation procedure for equivalent series capacitance C_se, ESR R_se, voltage ripples V, and RMS currents I_k in the capacitors is:

• Calculate reactances of individual capacitances according to Equation 4.

• Determine equivalent parallel parameters C_pk, R_pk of the capacitors based on Equations 2 and 3.

• Calculate equivalent parallel capacitance C_pe of the structure, its reactance X_pe, and equivalent parallel resistance R_pe  according to Equations 5, 9, and 6.

• Calculate equivalent series capacitance C_se and ESR R_se of the structure according to Equations 7 and 8.

• Obtain RMS ripple voltage V using Equation 1.

• Calculate RMS currents I_k in the capacitors based on:

Note that ESR values R_sk are strong functions of frequency. A designer should use ESR data specified by capacitor manufacturers at a given frequency of operation, such as the data for ceramic and polymer aluminum electrolytic capacitors from Murata Manufacturing Co. Ltd. (MMC) (http://ds.murata.co.jp/software/simsurfing/en-us/index.html).

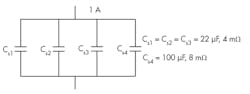
To illustrate the calculation procedure, let’s determine equivalent parameters, voltage ripple, and current distribution for a parallel connection of three ceramic capacitors (GRM21BR60J226ME39L) and one polymer capacitor (ESASD40J107M015K00) from MMC (Fig. 2).

Using the data f = 200 kHz, C_s1 = C_s2 = C_s3 = 22 μF, R_s1 = R_s2 = R_s3 = 4 mΩ, C_s4 = 100 μF, R_s4 =8 mΩ, I = 2 A, then:

• For reactance of each individual capacitance according to Equation 4, we have X_si = X_s2 = X_s3 = 3.6 mΩ, X_s4 = 0.8 mΩ.

• Equivalent parallel parameters C_pk, R_pk of the capacitors based on Equations 2 and 3 are C_p1 =C_p2 = C_p3 = 21.7 μF,  R_p1 =R_p2 = R_p3 = 331 mΩ, C_p4 = 49.7 μF, R_p4 = 16 mΩ.

• For equivalent parallel capacitance C_pe, its reactance X_pe and equivalent parallel resistance R_pe of the structure according to Equations 5, 9, and 6, we calculate C_pe = 115 μF, X_pe = 6.9 mΩ, R_pe = 13.9 mΩ.

• According to Equations 7 and 8, the equivalent series capacitance C_se and ESR R_se are C_se = 143.4 μF, R_se = 2.76 mΩ. 

• For RMS ripple voltage V based on Equation 1, we obtain V = 12.4 mV.

• RMS currents according to Equation 10 in ceramic and polymer capacitors are respectively: I₁ = I₂ = I₃ = 341 mA, I₄ = 1.1 A.

This shows the technique can easily determine the parameter values in each of the capacitors.

Alexander Asinovski is principal engineer at Murata Power Solutions Inc., Mansfield, Mass. He holds BSEE and MSEE degrees from State Technical University, St. Petersburg, Russia, and a PhD from the University of Telecommunications, St. Petersburg. He can be reached at [email protected].