Copper Alloy Inductors Stabilize Current Sensing

Current sensing is a critical part of microprocessor VR11x computing power as it provides overcurrent protection, phase-to-phase balancing and load line adherence. The trend is to lower voltage and improve efficiency in the power architecture. This requires tighter voltage regulation during transients with the accuracy of current sensing being critical.

Recommended current-sensing methods vary among vendors, but sensing through an inductor's resistance (R_DC) is a popular method because it is considered lossless. Less than 1% is degraded from the power efficiency, and accuracy is good because the inductor is sensing the actual output current to the load. The stability of the inductor's inductance and R_DC over temperature is critical, and tighter tolerances can improve the accuracy of the measurement.

Presently, negative temperature coefficient of resistance (NTC) thermistors are used to sense the inductor's temperature. The thermistor provides correction for the change in resistance of the inductor's copper conductor material, which has a large temperature coefficient of resistance (TCR), 3900 ppm/°C.

However, a thermistor is an additional component on the bill of materials; it complicates the board layout and is not dynamically responsive. By using a copper-alloy material for the inductor conductor, the TCR can be lowered to 700 ppm/°C, and the tolerance at room temperature can be controlled to ±2%, essentially making for an inductor with a constant R_DC.

Thermal Correction with an NTC Thermistor

A closer examination of how an NTC thermistor is used in current sensing can show why it has a large error margin due to nonuniform heat distribution.

Current is sensed across the inductor in each phase of the voltage regulator by detecting the voltage across the inductor's R_DC . An R-C network is connected in parallel to the inductor where the voltage across the capacitor is proportional to the inductor output current. If the time constant of the R-C network matches the time constant of the inductor, L/R_DC , then accurate sensing is accomplished.^[1]

Compensating for the high TCR of the copper-inductor conductor requires a thermal sensor. Thermal sensing is typically done external to the PWM control chip, because the chip is not positioned next to the components that are dissipating high amounts of heat.

An NTC thermistor is located close to one of the output inductors along with a biasing resistor. The number of output inductors depends on the number of voltage regulator phases. Errors in the compensation method occur because there are differences in board and component temperature and the thermistor can't dynamically respond. This error margin is most critical for output-voltage load line regulation.

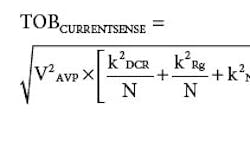
According to STMicroelectronics' data sheet for the L6756 multiphase controller for VR11x applications^[2], three factors typically affect the load line regulation tolerance band: controller tolerance, current-sense circuit tolerance and time-constant matching error tolerance. The tolerance band for the current-sense circuit is directly related to the inductor DCR tolerance, the accuracy of the NTC thermistor and the accuracy of the temperature measurement for the copper-inductor conductor. The formula for calculating this tolerance is:

where V_AVP is the output voltage (adaptive voltage positioning), k_DCR is the inductor R_DC tolerance, k_Rg is the trans-conductance resistor, k_NTC0 is the tolerance of the NTC thermistor at room temperature, á is the copper temperature coefficient of resistance, k_NTC is the temperature accuracy, ΔT is the change in temperature, DCR is the inductor R_DCat room temperature and N is the number of phases.

The error related to the inductor R_DC tolerance can be divided by the number of phases as can the error for the Rg resistors. This helps reduce the significance of these variables. However, the tolerance on the NTC thermistor and the error in temperature measurement are not impacted by the number of phases. These two variables are significant factors in the accuracy of the sensing. If the thermistor is eliminated and the coefficient of resistance is minimized by the selection of the conductor material, then the error associated with the inductor R_DC current sensing can be improved significantly.

Traditionally, copper has been used for the conductor material in inductors because of its very low resistance, which provides a small physical conductor size. The dc resistance is a measure of a material's resistance to the flow of electric current and is defined as:

where ρ is resistivity (Ω-m), R is resistance (Ω), A is cross-sectional area (m²) and l is length (m).

The table shows a resistivity comparison between several common materials. Like all things in engineering, tradeoff is the name of the game. And if we can obtain a material with a slightly higher resistivity but with a much better TCR, then there's a net advantage. As can be seen from the table, a resistive alloy with a very low TCR is a good choice.

TCR defines the amount of change in a material's resistance over a change in temperature (°C) expressed in percent or parts per million. At normal operating circuit conditions, the electric resistance of metal conductors varies linearly with temperature. Most metals increase in resistivity as temperature increases. Copper typically increases 0.39%, or 3900 ppm. For example, a 1-mΩ part becomes 1.4 mΩ for a 100°C change in temperature.

Resistive alloys have a much more stable TCR compared to copper. There are many different alloy materials, but one with a TCR of 0.07%, or 700 ppm, is an excellent choice for a lossless inductor. A conductor of this material with an RDC of 1 mΩ would equate to 1.07 mΩ for the same conditions noted previously. The tradeoff for the resistive alloy is that, due to a higher resistivity level, the conductor must be physically larger to achieve a low resistance when compared to copper. When evaluating R_DC at maximum operating temperature conditions, the difference is not as significant.

A comparison of single-turn conductor strips that equate to the same R_DC at 125°C is shown in Fig. 1. The larger conductor on the left is with the resistive alloy material and the smaller conductor on the right is with copper. To minimize the difference in the physical size of the conductor, the alloy width and thickness were doubled while the length was held constant.

The overall impact on the physical size of the inductor is shown to scale in Fig. 2. The inductor with the copper conductor is approximately 7 mm × 10 mm while the inductor with the alloy conductor is approximately 10 mm × 11 mm. The overall height and length are similar with the main difference being the width of the part.

Even though this size difference is a negative, the gain in temperature stability is considerable. Fig. 3 shows how a copper conductor that starts at a R_DC of 0.50 mΩ at 25°C will increase to 0.74 mΩ at 125°C while the alloy material will increase from 0.60 mΩ at 25°C to 0.64 mΩ at 125°C. In actual circuit conditions, the 125°C is not unrealistic as part of the normal operating parameters.

If you take into consideration that the nominal R_DC of the resistive alloy material will be toleranced at ±2% instead of the inductor industry standard of ±8% for copper, the tighter tolerance band over temperature is significantly better. Fig. 4 shows again the 0.5-mΩ copper conductor versus the 0.6-mΩ alloy conductor, but with the tolerance band also shown. The copper conductor, when selected at the low and high end of the tolerance band over temperature, can vary from 0.46 mΩ to 0.79 mΩ while the alloy conductor can vary from 0.59 mΩ to 0.66 mΩ.

There are many challenges an inductor manufacturer encounters when controlling the R_DC precision on the production floor. The ability to accurately measure is easier to accomplish with a resistive alloy than with copper because of the lower temperature sensitivity of the alloy. If the temperature of the copper conductor is just 1°C higher than previous test conditions, the resistance will change by 0.39%. With the resistive alloy material, a 1°C change in temperature is only a 0.07% change in value, much less significant in the manufacturing test environment.

In addition, the low ohmic range offers a significant engineering challenge with regard to repeatability. This has two main facets. First, the mechanical geometry of the part must be tightly controlled. The conductor must not have a stack up of greater than ±1% in any dimension, which equates to 0.000118 in. (0.003 mm) in thickness, 0.0068 in. (0.175 mm) in length or 0.002 in. (0.05 mm) in width.

The second facet is the low and precise resistance value that the above geometry entails. The conductor must have a very consistent 2% tolerance to perform the task, which is equivalent to 20 µΩ of variation from a target resistance of 1 mΩ with a resistive alloy. In applications that use copper, the challenge increases further by the even lower resistance values. If a copper conductor is used, then at a resistance of 0.3 mΩ, a 2% part would only permit 6 µΩ of variation.

Simple ohm-meters will not accurately measure resistance at these small levels because of the voltage drop in the measuring leads; four-terminal sensing is required. Clearly defined points of measurement must be identified on the conductor (Fig. 5). A four-terminal connection increases the accuracy of the current measurement by removing the resistance in the test leads and solder connections.

Typically, a solder connection is considered to have no appreciable impact on the overall resistance. But it plays a larger role in the situation of a low-resistance current-detection shunt at very low values. At high currents and low-resistance values, even the smallest amount of differences in resistance will affect signal accuracy. For example, a solder joint that added 5 µΩ of resistance would introduce an additional 1% of error on a 1% resistor at 0.5 mΩ.

Two terminal connections would introduce an excessive amount of error at the solder-joint region mentioned previously and the additional lead length from the part. This type of application requires a four-terminal connection often referred to as a Kelvin connection, which connects two points for a current path and two points to measure the voltage signal. This results in a higher-precision resistance measurement by removing the voltage drop that would occur due to the contact resistance. This method for measurement must be used for these low ohmic values due to the sensitive nature of these measurements.

Additionally, these measurements must be made continuously and fed back to the equipment during the manufacturing process to assure the precision of the part throughout the production. If this feedback method were not used, the precision would be entirely dependent on the absolute consistency of the mechanical dimensions of the conductor material from the mill.

Using a new and innovative inductor conductor material and improved manufacturing process with test capabilities can provide a precise current-sensing inductor with a tight tolerance band and temperature-stable current sensing. This device will aid PWM controller manufacturers in their quest for a higher level of control accuracy for multiphase voltage regulators.

References

1. Huang, Wenkang; Clarkin, John; Cheng, Peter; and Schuellein, George. “Inductors Allow Loss-Less Current Sensing in Multiphase DC-DC Converters,” PCIM magazine, June 2001.

2. “L6756 2/3/4 Phase Buck Controller for Processor Applications,” STMicroelectronics data sheet, February 2008.